Example

Example

Suppose that a source s must assess with what degree it is possible, in its opinion, that a pixel belongs to a set of three classes.

Let

d_s¹(x) = 0.7 the degree allotted to class 1,
d_s²(x) = 0.3 the degree allotted to class 2,
d_s³(x) = 0.5 the degree allotted to class 3.

We get $\sum_{c=1}^3 d_s^c(x) = 1.5$ .

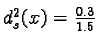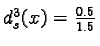First, we assign the masses of evidence m_s^c so that their sum equals 1, and so $m_s^1(x) = \frac{d_s^1(x)}{\sum_{c=1}^3 d_s^c(x)} = \frac{0.7}{1.5}$ , $d_s^2(x) = \frac{0.3}{1.5}$ , et $d_s^3(x) = \frac{0.5}{1.5}$ .
We must now determine what is the uncertainty of this source s about the choice of the class to which pixel x belongs.

[Zahzah, 1992] proposed to evaluate this uncertainty $\theta$ by the distance between the class which is considered to be the most plausible for pixel x, and all the other classes. This distance is likened to the degree of confusion between the classes which are possible for this pixel.

Two situations can be considered:

1.: If the source indicates:
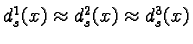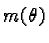2.: At the opposite, we can be in a situation of high confusion. The source ignores which class must be given more importance and then assigns close degrees( $d_s^1(x) \approx d_s^2(x) \approx d_s^3(x)$ ) to each class. In this case, confusion is very high. To express this confusion, a large part of the mass of evidence is assigned to $m(\theta)$ .

IRIT-UPS