Comparison between possibility and probability

Comparison between Possibility and Probability

Possibility and necessity measures, like probability measures, constitute tools to represent uncertainty.

Their properties are different, but they are however comparable. In the same way, the properties of possibility distributions can be compared with the properties of probability distributions:

probability measures summarize a body of precise and varied knowledge, while
possibility measures reflect a vague but coherent knowledge.

The possibility functions are more natural for the representation of the feeling of incertitude: very precise information is not expected absolutely from someone, but we do hope for the greatest possible coherence in his remarks. On the other hand, precise but fluctuating data more usually result from the observation of a physical phenomenon.

Probability on the one hand, and possibility-necessity on the other hand, correspond to two extreme, therefore ideal situations.

Table 17 shows the main points of comparison.

It can be seen that the identities have a similar form. The differences arise from the fact that

any addition in probability theory is replaced, in possibility theory, by a maximum, and that
any product is replaced by a minimum.

Identical properties are subjected to fewer constraints in possibility theory than in probability theory. Moreover, the values of measures and distributions are selected with more freedom in the case of possibility than in the case of probability.

**Table 17:** *Comparison of the main formulae of **possibility** theory with those of **probability** theory.*
Possibility	Probability
Distribution $\pi$ , measure $\Pi$	Distribution p, probability P
$\sup_{x \in \Omega} \pi(x) = 1$	$\sum_{x \in \Omega} p(x) = 1$
$\forall (A, B) \in \mathcal{P}(\Omega)^2$	$\forall (A, B) \in \mathcal{P}(\Omega)^2$

$\Pi(A) = \sup_{x \in A} \pi(x)$	$P(A) = \sum_{x \in A} p(x)$

$\Pi(A \cup B) = \max(\Pi(A), \Pi(B))$	$P(A \cup B) = P(A) + P(B)$ si $A \cap B = \varnothing$
$N(A \cap B) = \min(N(A), N(B))$	$P(A \cap B) = P(A) \cdot P(B)$ if independent

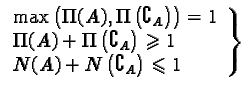$\left. \begin{array}{l}\max\left(\Pi(A), \Pi\left(\complement_A\right)\right)......lant 1 \\N(A) + N\left(\complement_A\right) \leqslant 1\end{array}\right\}$	$P(A) + P\left(\complement_A\right) = 1$

Ignorance: $\forall x \in \Omega, \quad \pi(x) = 1$	Ignorance: $\forall x \in \Omega, \quad p(x) = \frac{1}{\vert\Omega\vert}$

IRIT-UPS